
Many different types of patterns occur in the Game of Life, including static patterns (" still lifes"), repeating patterns (" oscillators" – a superset of still lifes), and patterns that translate themselves across the board (" spaceships"). Settling into a stable configuration that remains unchanged thereafter, or entering an oscillating phase in which they repeat an endless cycle of two or more periods.Fading away completely (from overcrowding or from becoming too sparse) or.There should be simple initial patterns that grow and change for a considerable period of time before coming to an end in the following possible ways:.
There should be initial patterns that apparently do grow without limit.There should be no initial pattern for which there is a simple proof that the population can grow without limit.It developed a cult following through the 1970s and beyond current developments have gone so far as to create theoretic emulations of computer systems within the confines of a Life board.Ĭonway chose his rules carefully, after considerable experimentation, to meet three criteria: For some, however, Life had more philosophical connotations. For many, Life was simply a programming challenge a fun way to waste CPU cycles. In this respect it foreshadowed the later popularity of computer-generated fractals.
The popularity of Conway's Life was helped by its coming into being just in time for a new generation of inexpensive minicomputers which were being released into the market, meaning that the game could be run for hours on these machines which were otherwise unused at night.
#Conways game of life command line output free#
Dennett has used the analog of Conway's Life "universe" extensively to illustrate the possible evolution of complex philosophical constructs, such as consciousness and free will, from the relatively simple set of deterministic physical laws governing our own universe. For example, philosopher and cognitive scientist Daniel C. The game can also serve as a didactic analogy, used to convey the somewhat counterintuitive notion that "design" and "organization" can spontaneously emerge in the absence of a designer. It is interesting for physicists, biologists, economists, mathematicians, philosophers, generative scientists and others to observe the way that complex patterns can emerge from the implementation of very simple rules. Life is an example of emergence and self-organization. Because of Life's analogies with the rise, fall and alterations of a society of living organisms, it belongs to a growing class of what are called 'simulation games' (games that resemble real life processes)Įver since its publication, Conway's Game of Life has attracted much interest because of the surprising ways in which the patterns can evolve.

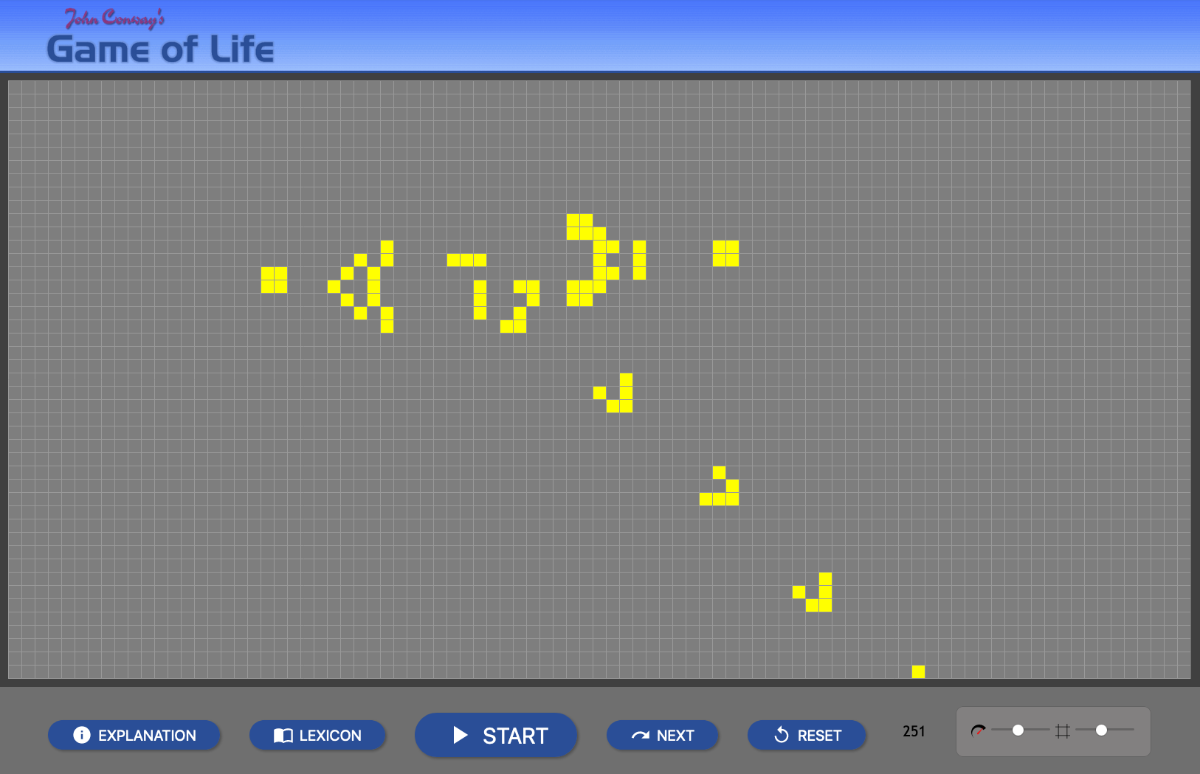
The game made Conway instantly famous, but it also opened up a whole new field of mathematical research, the field of cellular automata. From a theoretical point of view, it is interesting because it has the power of a universal Turing machine: that is, anything that can be computed algorithmically can be computed within Conway's Game of Life. The game made its first public appearance in the October 1970 issue of Scientific American, in Martin Gardner's "Mathematical Games" column, under the title of The fantastic combinations of John Conway's new solitaire game "life". The Game of Life emerged as Conway's successful attempt to simplify von Neumann's ideas. (In other words, each generation is a pure function of the one before.) The rules continue to be applied repeatedly to create further generations.Ĭonway was interested in a problem presented in the 1940s by renowned mathematician John von Neumann, who tried to find a hypothetical machine that could build copies of itself and succeeded when he found a mathematical model for such a machine with very complicated rules on a rectangular grid. The first generation is created by applying the above rules simultaneously to every cell in the seed - births and deaths happen simultaneously, and the discrete moment at which this happens is sometimes called a tick. The initial pattern constitutes the 'seed' of the system. Please enable Javascript to view this LifeViewer.Īnimated evolution of a pattern known as the two-glider octomino, with highlighted envelope (cells that were alive at some earlier point)


 0 kommentar(er)
0 kommentar(er)
